Whether a physics student, a science enthusiast, or even an engineer who deals with light, one definetely would have heard the word “wavenumber” being mentioned once in his way. But what does that term mean, and why should one take an interest in it? It is time to delve deep into this mystery idea, dig up the importance it carries, and find out why it plays such a vital role in the world of optics, waves, and light.
In other words, the wavenumber is the number of wave cycles in any given linear unit of distance. As a matter of fact, much of this had more to do with counting ripples on the pond, except this time it would deal with with light or sound waves navigating through space.
What is Wavenumber?
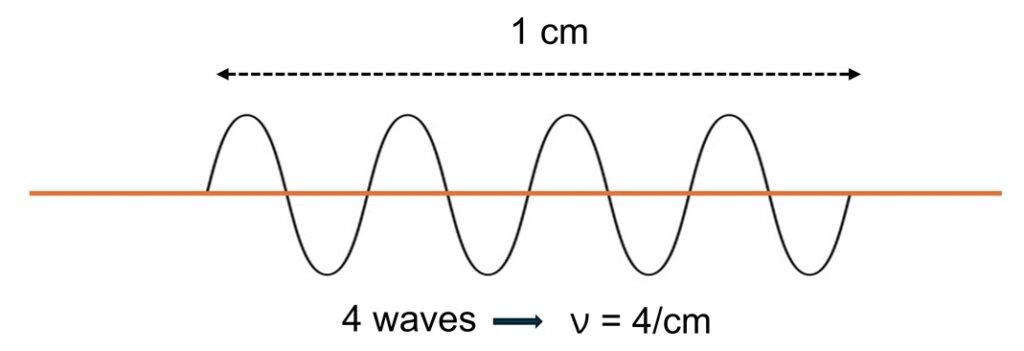
Wavenumber, often written as ν, represents the number of wavelengths that could fit into a given distance. It is commonly expressed in units of reciprocal meters (m⁻¹) or inverse centimeters (cm⁻¹). The wavenumber states how many times a wave vibrates up and down along in a particular length. This is very much connected with the wavelength and frequency of the wave. A visual representation is given in the image below.
Wavelength is the distance between two successive wave peaks. Frequency is the number of waves passing a given point every second. Wavenumber is something connected with both of these measures and is a quantity often used in spectroscopy, among other fields when one must be very precise with have analysis.
Why Wavenumber Matters
Wavenumber is not an abstract concept; it refers directly to real-life applications, especially in spectroscopy and laser technology, in understanding the behavior of light.
In infrared and Raman spectroscopy; wavenumber is one of the basic measured used in identifying moleculat vibration since different molecule will vibrate at characteristic wavenumbers, hence making it invaluable in chemical analysis.
In laser technology, wavenumber helps determine the color (or frequency) of light produced by the laser source. The wavenumber is essential in specifying the exact operating conditions of laser systems.
In material science, scientists use wavenumbers to study materials at a microscopic level by analyzing how different materials interact with light at various wavenumbers, they can learn about the material’s structure and properties.
How to Calculate Wavenumber?
You must have heard that “wavelength is the key” to determine the wavenumber; well, since we have said this above that the wavenumber is just the other side of the wavelength coin, what about frequency? Here is the equations that you can use to calculate wavenumber either by wavelength or frequency of light. These formulas will get you the wavenumber for any given wave. But remember, the units must be consistent. For example, if you use meters for the wavelength, your wavenumber will be in meters⁻¹.
$$\text{Wavenumber} \, (\tilde{\nu}) = \frac{1}{\text{Wavelength} \, (\lambda)}$$
$$\text{Wavenumber} \, (\tilde{\nu}) = \frac{\text{Frequency} \, (f)}{\text{Speed of light} \, (c)}$$
If you would like to convert between wavelength and wavenumber, you could use our wavelength to wavenumber or wavenumber to wavelength calculators.
Angular Wavenumber in Physics
The angular wavenumber is the most important constant in order to describe waves in three dimensions concerning wavee physics. It is related to the generalized number wavenumber but it brings added information information mainly about the angular frequency of the wave and how the waves propagate through different types of media. The angular wavenumber (𝑘) is defined as the number of radians the wave oscillates per unit distance. Mathematically, it is given by:
$$k = \frac{2\pi}{\lambda}$$
where 𝑘 is the angular wavenumber (in radians per unit length), λ is the wavelength of the wave.
Angular wavenumber is also related to angular frequency (𝜔) and frequency (𝑓) of the wave. Using the formula:
$$\omega = v k$$
where, ω is the angular frequency (in radians per second), 𝑣 is the wave speed. From this, we can see how angular wavenumber 𝑘 k is directly tied to both the temporal and spatial properties of the wave.
Conclusion
Even though the wave number could be a minor technical part, it becomes necessary for us to understand the nature of light and the wave behavior. A wavenumber is used in both spectroscopy and laser technology for the assessment of wave characteristics and the investigation of their interactions with other materials. After all, it’s the heartbeat of every wave!
FAQs About Wavenumber
What is the unit of wavenumber?
The unit of wavenumber is typically given in reciprocal meters (m⁻¹) or inverse centimeters (cm⁻¹), depending on the field of study. This unit represents the number of wave cycles in one meter or centimeter, respectively.
How is wavenumber related to energy?
Wavenumber is directly proportional to energy in the context of photons. Higher wavenumbers correspond to higher energies. This relationship is described by the equation given below where the E is the energy of the photon, ℎ is Planck’s constant, and 𝑐 is the speed of light.
$$E = h c \tilde{\nu}$$
Can wavenumber be negative?
No, wavenumber cannot be negative. It represents a positive count of waves per unit distance, so it must always be positive.
Why do we use wavenumber instead of wavelength?
In many applications, especially in spectroscopy, wavenumber is more useful because it gives a more direct measurement of energy. The higher the wavenumber, the higher the energy, which is essential in many scientific fields like chemistry and physics.
Did you know?
– In quantum mechanics, wavenumber is a very important aspect in understanding the wave properties of particles. The de Broglie wavelength, which settles the argument of wave-particle duality, is defined as inversely proportional to the momentum of the particle. With the increase in particle’s momentum wavenumber increases also, thus enhancing our comprehended nature of matter within quantum level even further.
– Wavenumbers allow even those signals emanating from space to be analyzed-be it the light from distant stars or even radio waves from galaxies. In analyzing such signals, the astronomer is able to make out compositions, motions, and distances of an object. Actually, in observing cosmic background radiation that emanated from the Big Bang, scientists use wavenumber in trying to make sense of the early universe!
– Wavenumbers are highly relevant in medical diagnostics, especially in techniques such as infrared spectroscopy and MRI. The infrared region of the electromagnetic spectrum is where wavenumber ranges are applied to investigate the chemical nature of tissues in which pathologies, like tumors, may be recognized based on the identification of molecular vibrations. Similarly, wavenumbers are important for knowing tissue properties in MRI studies.